Mathematician (CV)

A picture of Abe.

Starting August 2015, I am an Assistant Professor at University of Wisconsin—Stout, Wisconsin's Polytechnic University.

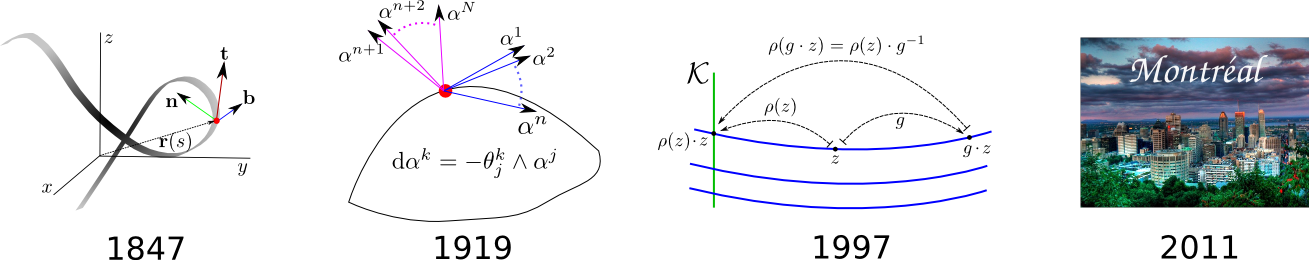
From 2011 until 2015, I was a Peter M. Curran Visiting Assistant Professor at Fordham University. Previously, I was a postdoctoral fellow at McGill University, mentored by Niky Kamran and funded by an NSF fellowship administered by the MSRI. I earned my PhD in 2009 at Duke University under the direction of Robert Bryant.

Networks

Teaching, Fall 2014

  • Math 3010

    Scientific Communications

    This is a course on scientific writing and publishing, and it provides credit for the Eloquentia Perfecta 3 requirement.
      Mondays and Thursdays, 10:00 to 11:15 (Block B) in room JMH 406.
      Assignments are found on the Course Calendar. To access it, you must be logged into your @Fordham.edu Google account.
      For this course, you will need a laptop. Any modern operating system will work, but you will need to install
    • MacTeX for Apple MacOS X
    • ProTeXt for Microsoft Windows
    • TeXlive for Linux and other UNIX/BSD systems (though it is usually better to install the version of texlive-full included in your Linux distribution).
      One very userful tool is a version control system. If you aren't already familiar with one, I suggest trying SourceTree. I won't require its use, but it will make your life easier.
      I put a short sequence of videos on YouTube about using PGP/GnuPG for message verification. This is not essential or required for our course, but I will sign all of the files I provide for you, so that you can be certain that they are uncorrupted when diagnosing problems. If you tell me “your example file didn't work,” the first thing I'll ask is “did you check the signature?”
    All files will be signed with 0x7ECF418F. GnuPG can be downloaded for On most Linux systems, GnuPG is preinstalled by default, as it is used to verify that the system packages are uncorrupted. This is how we maintain trust in open-source software.
  • Math 1000 Sect 4

    Pre-calculus

    This is a course on linear geometry, algebra, and trigonometry for those hoping to move into Math 1203 or 1206 next semester.
      Mondays and Thursdays, 16:00 to 17:15 (Block E) in room JMH 140.
      Assignments are found on the Course Calendar. To access it, you must be logged into your @Fordham.edu Google account.
      We will be using Fordham WebWork for online homework.

  • Help Room

    The Math Help Room (JMH 410) is a great resource! Feel free to use the Help Room any time. Every teacher in the department has a shift, and someone will be there who can help.
      My shift in Fall 2014 is 12:30--13:30 on Mondays and Thursdays.
    My Office Hours in Fall 2014 are 11:30--12:30 on Mondays and Thursdays.

Teaching, Spring 2014

  • Linear Algebra II - Math 3001 Section 1. Mondays and Thursdays, 10:00 to 11:15 (Block B) in JMH room 406.
  • Applied Calculus I - Math 1203 Section 1. Mondays and Thursdays, 08:30 to 9:45 (Block A) in Freeman Hall room 108.
  • Help-Room Hours: 12:30--13:30 and 14:30--15:30 Mondays.

Teaching, Historical

Fall 2013

  • Pre-calculus - Math 1000 Section 4. Mondays and Thursdays, 10:00 to 11:15 (Block B) in room JMH 140.
  • Scientific Communications - Math 3010 Section 1. Mondays and Thursdays, 11:30 to 12:45 (Block C) in room JMH 406. This is a course on scientific writing and publishing, and it provides credit for the Eloquentia Perfecta 3 requirement.
    I put a short sequence of videos on YouTube about using PGP/GPG for message verification. This is not essential or required for our course, but I will sign all of the files I provide for you, so that you can be certain that they are uncorrupted when diagnosing problems. If you tell me “your example file didn't work,” the first thing I'll say is “did you check the signature?”

Spring 2013

  • Finite Mathematics - Math 1100 Section 1. Mondays and Thursdays, 8:30 to 9:45 in room JMH 404.
  • Finite Mathematics - Math 1100 Section 2. Mondays and Thursdays, 10:00 to 11:15 in room JMH 404.
  • Linear Algebra I - Math 2006 Section 1. Mondays and Thursdays, 11:30 to 12:45 in room JMH 406.
    Our textbook is by Hefferon. My end-of-semester notes are available in paper and tablet formats. These are works-in-progress.
  • Help-Room Hours: Mondays, 13:30 to 15:30 in room JMH 410.
  • Office Hours: Thursdays, 13:30 to 15:30 in room JMH 422B.

Fall 2012

  • Pre-calculus - Math 1000 Section 1. Mondays and Thursdays, 8:30 to 9:45 in room JMH 342.
  • Differential Geometry - Math 4020. Mondays and Thursdays, 10:00 to 11:15 in room JMH 406.
  • Help-Room Hours: Mondays 13:30--15:30.
  • Office Hours: Thursdays 12:30--14:30, or email me to arrange a time.

Summer 2012 (Session 2, July 2 -- August 7)

  • Pre-calculus - Math 1000 Section 21. Tuesdays, Wednesdays, and Thursdays from 09:00 to 12:00 in room JMH 406. NOTE: July 7 through July 13, class will be held in Dealy 302.

Spring 2012

  • Multivariable Calculus I - Math 2004 Section 1. Mondays and Thursdays from 14:30 to 15:45.
  • Calculus II - Math 1207 Section 2. Mondays and Thursdays from 16:00 to 17:15.
  • Help-Room Hours: Mondays 11:30--13:30.
  • Office Hours: Wednesdays 13:00--14:00 and Thursdays 11:30--12:30.

Teaching, Fall 2011

  • Pre-calculus - Math 1000 Section 1, Block A on Mondays and Thursdays from 8:30 to 9:45 in Room JMH 132.
  • Calculus I - Math 1206 Section 2, Block B on Mondays and Thursdays from 10:00 to 11:15 in Room JMH 132.
  • Calculus I - Math 1206 Recitation Sections 1, 4, and 5. Tuesdays.
  • Help-Room Hours: Mondays 12:30--14:30.
  • Office Hours: Thursdays 13:00--15:00.

Teaching Evaluations

  • Fall 2013

    Scientific Communications

     A course on scientific and technical writing, for 3rd year science majors.
    Students learn LaTeX and prepare presentations and detailed scientific review articles on topics of their choice.
  • Spring 2012

    Calculus 2

    A typical second-semester Calculus course on integration, sequences, and series. We used Stewart's textbook.
    Note that responses are significantly better than the departmental average!
  • Fall 2012

    Differential Geometry

     An advanced elective on curves, surfaces, Riemannian geometry, and a little bit of cosmology.
  • Fall 2012

    Precalculus

    An introductory course to prepare freshmen for Calculus.
    Covering the algebra of functions.
    Polynomials, exponentials, logarithms, and a bit of trigonometry.

Research

I study geometry in the sense of E. Cartan; this means that I examine the intrinsic geometry of PDEs using exterior differential systems, moving frames and representation theory.

In particular, I am currently studying the phenomena of involutivity and hydrodynamic integrability using Guillemin normal form and Spencer cohomology. These tools are algebraic tools developed during the 1960s to study formal integrability criteria. Nowadays these formal methods are particularly useful, because they allow us to study PDEs using computer algebra software.

Alongside my theoretical work, I am writing an open-source package for Sage to automatically build, manipulate, classify, and solve PDEs and differential ideals.

Additionally, I am interested in the related frameworks for studying algebroids, pseudo-groups, and distributions. Control theory, mathematical relativity and Finsler geometry are neat, too.

On Dec 9, 2013, I gave a talk about my ongoing work at the Fields Institute in Toronto, as part of the Focused Research Workshop on Exterior Differential Systems and Lie Theory. You can watch a video of the talk to get a sense of my current project. (Be forewarned that my travel schedule meant it was my least-prepared talk ever.)

Science and Technology

Beyond this particular specialty, my interest in science is quite broad, and I try to stay abreast of developments in many branches of science. I try to read the weekly issues of Science and Nature, but I've also found that podcasts are an amazingly efficient way to learn new ideas. If you also have a general interest in science, I recommend these resources, which I follow religiously:

Source News Podcast Topic
RadioLab blog podcast Awesome presentation of contemporary science and the boundaries of human knowledge, for a general audience.
The Guardian's Science Weekly news podcast The Guardian's science reporters talk with experts in science and science policy. This is a nice median between the popular discussion of RadioLab and the technical intricacies of the Nature and AAAS/Science podcasts.
Nature News news podcast Newest articles from probably the most prestigious journal in the world. Excellent podcast!
Science/AAAS news podcast Newest articles from probably the second most prestigious journal in the world. Excellent podcast and good coverage of AAAS meetings.
Inside Science info podcast BBC Radio 4's new weekly science show, with Adam Rutherford. Discussions focus on the life of working scientists today.
Material World blog podcast BBC Radio 4's old weekly general science show, with Quentin Cooper. Refreshingly sophisticated compared to a science show that airs on Fridays in the US.
Planet Money blog podcast NPR's great production analyzing modern global economics in a thoughtful and reasonably scientific way. Unfortunately, most of the content never reaches the airwaves; you have to follow the podcast.
Relatively Prime info podcast A really thoughtful project outlining the role of modern research mathematics.
DisasterCast info podcast A fascinating series about systems engineering and safety.

Additionally, aside from their good coverage of consumer technology and Internet policy, Ars Technica has a fantastic science blog known as The Scientific Method.

The most insightful commentaries I have seen about research are The Importance of Stupidity in Scientific Research by Martin A. Schwartz and You and Your Research by Richard Hamming. Regarding mathematics as a social activity, everyone should read On Proof and Progress in Mathematics and this short essay by William Thurston. On a lighter note, a fantastic description of how it feels to do mathematics was recently written by Yasha Berchenko-Kogan.

Recently, I've also started using Twitter to follow other scientists and science journalists.

CMS Special Session, June 2013

Francis Valiquette and I organized a special session on Pseudogroups at the 2013 Summer Meeting of the Canadian Mathematical Society in Halifax.

CRM Workshop, June 2011

Francis Valiquette and I organized the workshop Moving Frames in Geometry at the Centre de Recherches Mathématiques at Université de Montréal. Talk slides are now available.
The classification of connected 2,3-integrable GL(2)-structures of degree 4. which correspond to Hessian-type integrable hydrodynamic PDEs in three variables.

Publications and Preprints

The classification of connected 2,3-integrable GL(2)-structures of degree 4. which correspond to Hessian-type integrable hydrodynamic PDEs in three variables. The classification of connected 2,3-integrable GL(2)-structures of degree 4 as well as Hessian-type integrable PDEs in three variables.

Preprints (Live)

Abraham Smith's articles on arXiv

[1]  http://arxiv.org/abs/1410.6947v2 [pdf]
Degeneracy of the Characteristic Variety
Abraham D. Smith
Subjects: Analysis of PDEs (math.AP); 58A15, 35A27

The characteristic variety plays an important role in the analysis of the solution space of partial differential equations and exterior differential systems. This article studies the linear span of this variety, measuring its dimension via an integrable extension of the original system. In the PDE case with locally constant characteristic variety, this extension yields a recursive version of Guillemin normal form, inducing a sequence of foliations on integral manifolds.

[2]  http://arxiv.org/abs/1410.7593v1 [pdf]
Constructing Involutive Tableaux with Guillemin Normal Form
Abraham D. Smith
Subjects: Analysis of PDEs (math.AP); 58A15, 58H10

Involutivity is the formal algebraic property that guarantees solutions to an analytic and torsion-free exterior differential system or partial differential equation via the Cartan--K\"ahler theorem. Guillemin normal form characterizes involutive systems by an almost-commuting property of the symbol map on certain subspaces of the prolongation's tableau. This article examines Guillemin normal form in detail, aiming at a more systematic approach to classifying involutive systems. The main result is an explicit quadratic condition for involutivity of the type suggested but not completed in Chapter IV \S5 of the book \emph{Exterior Differential Systems} by Bryant, Chern, Gardner, Goldschmidt, and Griffiths. To show the utility of this condition, several classical facts regarding involutive tableaux and the characteristic variety are re-proven explicitly using elementary techniques. It is hoped that this approach will make the subject accessible to a wider population of researchers, including undergraduates.

[3]  http://arxiv.org/abs/1010.6010v1 [pdf]
A Geometry for Second-Order PDEs and their Integrability, Part I
Abraham D. Smith
Subjects: Differential Geometry (math.DG); Analysis of PDEs (math.AP); 58A15, 37K10

For the purpose of understanding second-order scalar PDEs and their hydrodynamic integrability, we introduce G-structures that are induced on hypersurfaces of the space of symmetric matrices (interpreted as the fiber of second-order jet space) and are defined by non-degenerate scalar second-order-only (Hessian) PDEs in any number of variables. The fiber group is a conformal orthogonal group that acts on the space of independent variables, and it is a subgroup of the conformal orthogonal group for a semi-Riemannian metric that exists on the PDE. These G-structures are automatically compatible with the definition of hydrodynamic integrability, so they allow contact-invariant analysis of integrability via moving frames and the Cartan--Kaehler theorem. They directly generalize the GL(2)-structures that arise in the case of Hessian hyperbolic equations in three variables as well as several related geometries that appear in the literature on hydrodynamic integrability. Part I primarily discusses the motivation, the definition, and the solution to the equivalence problem, and Part II will discuss integrability in detail.

[4]  http://arxiv.org/abs/0912.2789v4 [pdf]
Integrable GL(2) Geometry and Hydrodynamic Partial Differential Equations
Abraham D. Smith
Subjects: Differential Geometry (math.DG); Analysis of PDEs (math.AP); Exactly Solvable and Integrable Systems (nlin.SI); 58A15, 37K10

This article is a local analysis of integrable GL(2)-structures of degree 4. A GL(2)-structure of degree n corresponds to a distribution of rational normal cones over a manifold M of dimension (n+1). Integrability corresponds to the existence of many submanifolds that are spanned by lines in the cones. These GL(2)-structures are important because they naturally arise from a certain family of second-order hyperbolic PDEs in three variables that are integrable via hydrodynamic reduction. Familiar examples include the wave equation, the first flow of the dKP equation, and the Boyer--Finley equation. The main results are a structure theorem for integrable GL(2)-structures, a classification for connected integrable GL(2)-structures, and an equivalence between local integrable GL(2)-structures and Hessian hydrodynamic hyperbolic PDEs in three variables. This yields natural geometric characterizations of the wave equation, the first flow of the dKP hierarchy, and several others. It also provides an intrinsic, coordinate-free infrastructure to describe a large class of hydrodynamic integrable systems in three variables.

Computing

Symbol for Sage

Have you ever wanted to build and manipulate differential ideals and symbols of PDEs on an open-source computer algebra system?

You're in luck! I'm developing >Symbol, a package for Sage. You can help at BitBucket.

Linux and Open-Source Software

Over time, I have spent an unreasonable number of hours learning the intricacies of Linux system administration. I have submitted patches and (properly documented) bug reports to various open-source programs, and I see the world of open-source software as another branch of the academic discipline. We all build on each-others' work, and it is scientifically unethical to rely on tools that you cannot ultimately deconstruct, understand, and modify.

Currently, for my own research, I am developing an Exterior Differential Systems package for Sage, the python-based, open-source math engine that is quickly taking over the world. My package will be modeled on Jeanne Clelland's excellent Cartan package for Maple, whose only problem is that it is a package for Maple.

My current and recent undergraduate research students have worked on problems in computer vision, including analyzing Spline curves in Randers geometry and developing moving-frame methods for image recognition and signal analysis.

Regarding Linux, I have used all the major distributions at one time or another, going back a long way, but for the moment I am settled on Debian. I recently found this, containing this disc and more, while going through an old box of books. I guess I really have been using linux a long time! (Actually, I think my brother bought that CD, but I do clearly recall running "make config" to build my kernel in RedHat 4.2 (Biltmore) in 1997.)

I have tinkered with things like IPv6 and other networking technologies. (I am curious what mathematical modeling and control theory can reveal about network catastrophes due to the Bufferbloat problem that has recently been brought to light.)

Contact Information

This page is valid HTML5 written by hand with Bootstrap.